Assignment 10:

Parametric Curves for Powers of Sine and Cosine

by

Jenny Johnson

What is a parametric curve?

A parametric curve in the plane is a pair of functions x = f(t) and y = g(t) where the two continuous function define ordered pairs (x, y). The two equations are called the parametric equations of a curve. The extent of the curve depends on the range of t.

We will explore the parametric curves when the parametric equations are different powers of cos(t) and sin(t).

What does the parametric curve of x = a cos (t) and y = b sin (t) look like?

For all of the following explorations, we will keep the range of t as 0 < t < 2π. First, let us look at the parametric curve when a = 1 and b = 1.

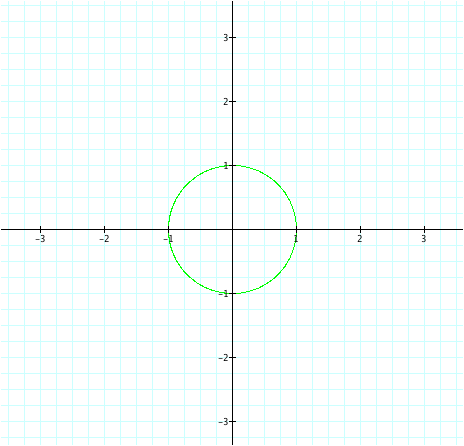
The graph is the unit circle! Now, what would the parametric look like if we had a =2 and b = 2.

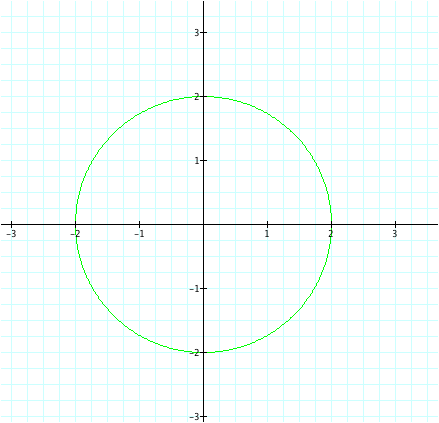
This parametric curve is a circle centered at the origin with a radius of 2. We could then make the conjecture that when a = b, the graph of the parametric curve will be a circle centered at the origin with radius of a (and b). Below is a movie of the parametric curves when a = b ranges from -5 to 5. Our conjecture that the graphs will be circles centered at the origin with r = a = b holds true.

Let us now explore the curve when we keep a = 1 and change the values of b. When b = 2, the curve looks like this:

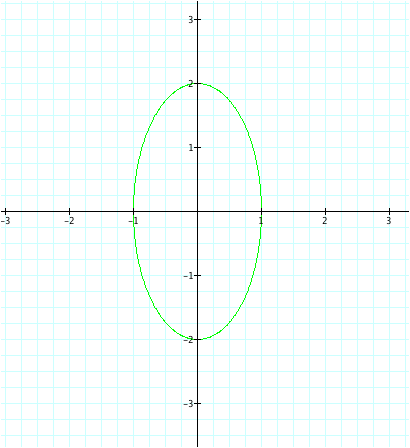
The graph is an ellipse. It appears that the value for a is the distance of the x-intercepts of the parametric curve from the origin and the value of b is the distance of the y-intercepts of the parametric curve from the origin. We can test this conjecture with the following movie with a = 1 and b values ranging from -5 to 5.

We see that when b is negative, the y-intercepts are a distance of |b| from the origin. Now, to test our conjecture of |a| being the distance of the x-intercepts from the origin, we will view a movie of the curve when b = 1 and the a values range from -5 to 5.

We can make the following observations when working with the parametric equations x = a cos (t) and y = sin (t):

1. When |a| = |b|, the parametric curve will be a circle centered at the origin with radius |a| (and also |b|).

2. When |a| > |b|, the parametric curve will be an ellipse with the x-axis as the major axis, the x-intercepts a distance |a| from the origin, and the y-intercepts a distance |b| from the origin.

3. When |a| < |b|, the parametric curve is an ellipse with the y-axis as the major axis, the x-intercepts a distance |a| from the origin, and the y-intercepts a distance |b| from the origin.

What does the parametric curve of x = a (cos (t))² and y = b (sin (t))² look like?

When a = 1 and b = 1, we get the following parametric curve.

We will graph this curve on the same axes as the parametric curve of x = a cos (t) and y = b cos (t) when a = 1 and b = 2.

It appears this curve will form a segment from the value of a on the x-axis to the value of b on the y-axis. The following movie shows both curves while a = 1 and b ranges from -5 to 5.

We can see that this new curve does form a line segment from the value of a on the x-axis to the value of b on the y-axis. We would then expect the line segment to be in Quadrant II when a is negative and b is positive, and in Quadrant III when a and b are negative. The following picture shows the curves when a = -3 and b = 2, and when a = -3 and b = -4, respectively.

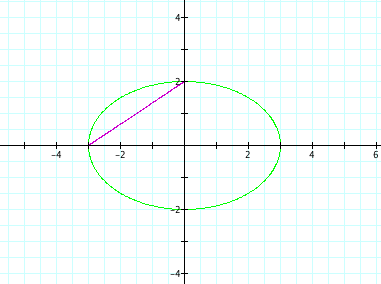
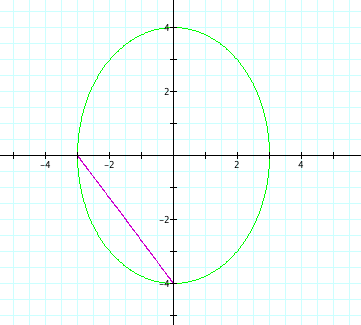
What does the parametric curve of x = a (cos (t))³ and y = b (sin (t))³ look like?

Let a = 1 and b = 1.

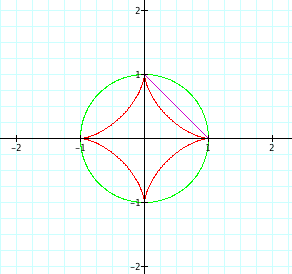
From the graph, we see that the x- and y-intercepts for the parametric curve of these parametric equations are the same as those for our first parametric equations. Let us see if this observation is the same when a = 2 and b = 5.

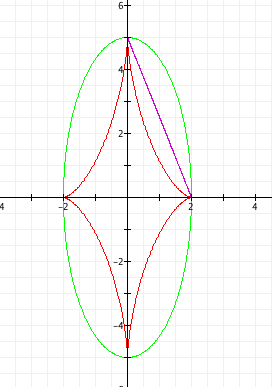
We see that the x-intercepts of this curve are a distance of |a| from the origin and the y-intercepts are a distance of |b| from the origin.

What does the parametric curve of x = a (cos (t))⁴ and y = b (sin (t))⁴ look like?

Starting with a = 1 and b = 1, we get the following graph.

We see this parametric curve is a curve from the value of a on the x-axis to the value of b on the y-axis. This is similar to the curve for x = a (cos (t))² and y = b (sin (t))² since it starts and ends at the same point, but it is a curve not a segment.

We can make the prediction that the curve of x = a (cos (t))⁵ and y = b (cos (t))⁵ will have x – intercepts of a and –a and y-intercepts of b and –b, but it will be closer to the pair x- and y-axis than the other curves (since the curves have come closer to the axes as the exponent for cosine and sine have increased). Let us see if our conjecture is true.

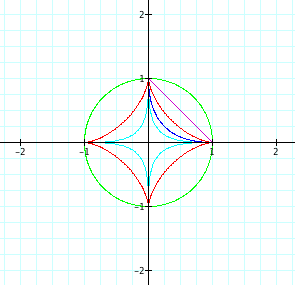
We are right! We can now make a few observations about the parametric curves based on the exponents for cos (t) and sin (t) in our parametric curves. The following conjectures are related to the parametric curves for the equations x = a (cos (t))ⁿ and y = b (sin (t))ⁿ.

1. When n is an odd number, the parametric curve will have two x-intercepts a and –a and y-intercepts b and –b.

2. When n is an even number, the parametric curve will only be in one quadrant and will be a segment or curve from (a, 0) to (0, b).

3. As n increases, the curve gets closer to the axes.

We can check our conjectures by graphing curves up to n = 9 on the same set of axes when a = 4 and b = -2.

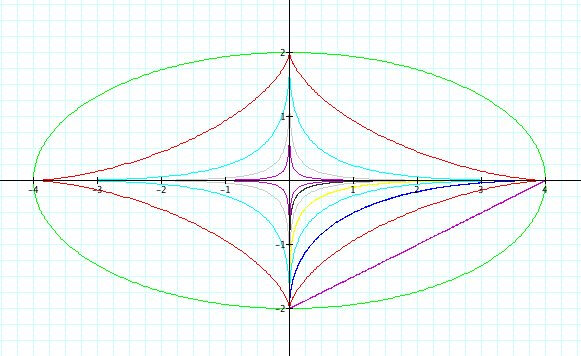
All of our conjectures hold true. Let us look at the following video with a = 4 and b values ranging from -5 to 5.

Return